Here you can find some useful information. Of note: this webpage is under construction. Thus, it is “constantly” evolving.

Teaching

Spring 2017 (Segundo Semestre 2017-2018)

Métodos cuantitativos para administración de empresas II: MECU 3032.

Courses taught during previous semesters can be found here

Research

Broadly, the research fields that currently are more appealling to me are (presentation about research):

  • Mathematical/computational models of complex systems.
  • Applied dynamical systems and bifurcation theory.
  • Power spectrum analysis and wavelets.
  • Parallel computing.
Computational models of complex systems

Many complex systems, e.g. biological systems and financial systems, can be described by differential equations. Such equations are mathematical objects that embody different principles or “laws of nature”. By describing the system with these equations and properly solving them, one can get an understanding of the inner workings of the system being considered. Moreover, math provides a precise language in which hypothesis about the system of interest can be formulated.

The following is a list of the complex systems that we study using mathematical models.

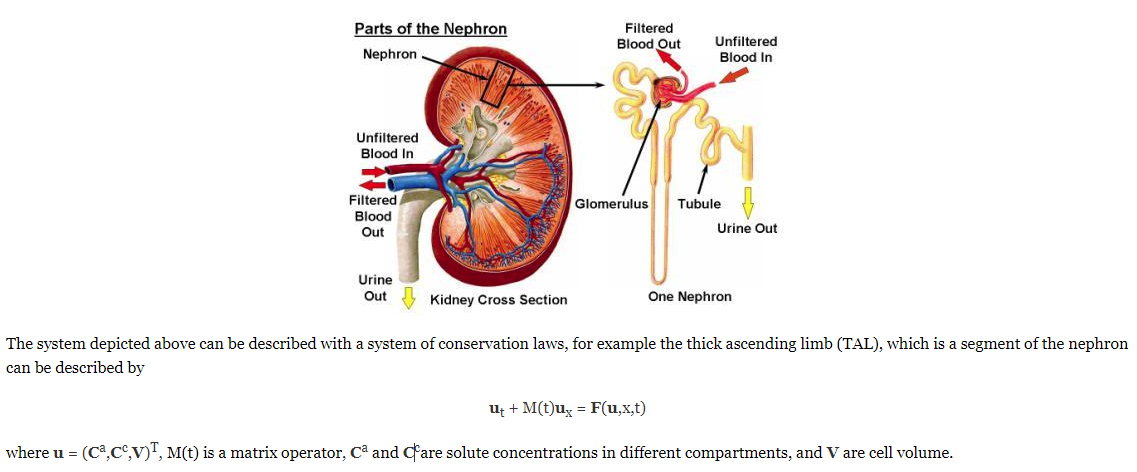
Human kidney

One of our project consists on modeling a segment of the nephron, called the Thick Ascending Limb (TAL) at the cellular and segmental level. The nephrons are tubules that are the functional unit of the kidney. This means that a nephron is the smallest part of that organ that carries out the functions of the organ as a whole (filtration, reabsorption, secretion and excretion). In particular the TAL segment is responsible of actively reabsorbing NaCl from the tubular fluid, hence it is a component of paramount importance that enhances the passive reabsorption of water that occurs across other segment of the nephron (collecting duct) whose outflow is the urine that eventually will get excreted from the human body. In addition, as one moves deeper into medullary regions of the kidney, TAL cells find themselves in a physiologically harsh environment in terms of osmotic challenges and oxygen availability. Thereby, through mathematical modeling our goal is to understand the different processes that occur in TAL cells, different aspects of their function including transport efficiency, and how the properties of these cells give rise to the physiological features of the segment as a whole.

The kidney, (renal physiology).
Renal physiology, image from  unckidneycenter.org.

Coral

Another project involves the development of a mathematical model of the population of coral polyps and its interactions with an unspecified pathogen and/or its environment. The model, besides being solved numerically, is going to be rigurously analyzed in order to gain insight of the physical system by studying its equilibria, and its bifurcations (local and global).

High frequency time series of stock prices

We study high frequency time series of stock prices using methods commonly used to analyze time series or time dependent signals in engineering and applied sciences. By high frequency, what is meant is that the elapsed time between subsequent observations is 1 minute or less. The autospectral, autocorrelation, and the coherence function is computed for each time series in order to characterize the time series in terms of periodicity, time delay, and linearity. Additionally we do a wavelet analysis of the time series. The wavelet analysis will allow us to detect transient oscillatory phenomena as well as to uncover phenomena within the time series that occurs at different time scales. All of the aforementioned analysis will allow us to better understand the mechanisms that underlie the system or process driving the changes in stock prices.

Parallel computing

Given the ubiquitous presence of multicore CPU’s in computer systems, from cell phones to supercomputers, it is advantageous to tap into the computational power that nowadays is readily available. Thereby, we are interested in the parellized implementation and development of numerical methods used to solve the models of the complex systems described above.

Interesting stuff to read, watch or listen to…

Disclaimer: for good or ill I, the author, is the sole responsible of this website and its contents.

Skip to content